Besides sports, I am also passionate about math. The passion started when I read the Chinese book 
(Intriguing Math) as a child. The book opened my eyes and made me realize that math is not something to be afraid of. It is actually a fascinating subject. I remember during a holiday celebration, my cousin and I walked to a local park in Beijing. There we found many math puzzles posted on large paper and spent a great deal of time solving the problems. It became a fond memory of our childhood.
My junior high math teacher, Mr. Sun, stoked my passion. During the Cultural Revolution in China, our classroom was chaotic. The rebellious students even threw coal balls while Mr. Sun was writing on the blackboard during a math class. I remember the bespectacled man with chalk dust over his clothes turned around and pleaded with them to stop. Amid chaos, Mr. Sun managed to show us that math is immensely interesting. It became my best subject. Once I got a perfect mark, 120 percent (with bonus points).
Later, I became a mother of four children. I have passed on my passion to them. Solving math puzzles is a family pastime for us. We challenge each other with math problems at the dinner table or on a car ride. When I studied to become a teacher at Ontario Institute for Studies in Education at the University of Toronto, math was a natural choice for my teachable subject. Since I got a job at the Toronto District School Board, I have shared my passion with students as well. In my spare time, I have also run a free math tutoring program for our neighbourhood kids.
While people collect stamps or baseball cards, I collect Terry Stickels’ math problems on the puzzles page of Toronto Star and bring them into classrooms. Last school year when I taught a Grade 8 class the algebra unit, I dug out related questions from my collection:
Example 1
For what value K, is the following system consistent?
1) P + Q = 6
2) KP + Q = 18
3) P + KQ = 30
Example 2
Let P, R, S and T be four whole, positive numbers (non-zero), such that three of the four following expressions are equivalent.
Which is the odd one out?
1) S/P = T/R
2) PT/SR = 1
3) P/S = R/T
4 ) P/T = R/S
Example 3
If A + B = Z
Z + P = T
T + A = F
B + P + F = 130
A = 20
What is the value of F?
Example 4
What is the smallest positive integer of K such that the product 396 × K is a perfect square?
Example 5
At a luncheon for physicians, all but 40 were neurosurgeons.
All but 50 were pediatricians, and all but 60 were cardiologists.
How many physicians were there in all?
Students seem to enjoy the questions which are not from the textbook. It is probably because the problems are a bit challenging and there is no repetition.
Here are two alphametic puzzles that I often use to challenge students.
Example 6
…THEY
…ARE
+ VERY
…SMART
This puzzle has two solutions. Remember, each letter equals a number and no word can begin with zero.
Example 7
Using the numbers 1 through 9 only once on the left-hand side of the equation, can you come up with three different positive fractions that add up to 1?
A/BC + D/EF+ G/HI = 1
BC, EF and HI are two-digit positive integers.
A, D and G are one-digit positive integers.
I usually give students this type of question as a mental set at the beginning of the class or to keep them occupied after they get their regular work done. Students love challenges. After they figure out the solutions, you can see the joy and pride on their faces. Kids challenge me too. Once a Grade 7 gifted boy at a middle school asked me how to calculate the area of an irregular shape, which led to a discussion of calculus.
I also got excellent questions from my math teacher at OISE, Mr. Cornwall, who won the Prime Minister’s Award in teaching mathematics and technology in 1994.
Example 8
There are three squares and eight rectangles. Their dimensions are as follows:
A: 4 × 25 = 100
B: 18 × 18 = 324
C: 10 ×15 = 150
D: 5 × 14 = 70
E: 6 × 13 = 78
F: 3 × 12 = 36
G: 9 × 11 = 99
H: 10 × 2 = 20
I: 7 × 8 = 56
J: 7 × 7 = 49
K: 2 × 2 = 4
Can you use these eleven shapes to form a new rectangle?
I like this question because it combines two strands, number sense and numeration (factors) and geometry (areas of a square and a rectangle). When I was struggling with it, my 8-year-old son said to me, “There are more probabilities.” That was a light bulb moment. Shortly afterwards, I put all pieces together. I was pretty happy about it.
Example 9
If you know how to use the “magic cards” below, you can ask a friend to think of a secret number between 1 and 31. Then ask your friend to answer “yes” or “no” to each of the following questions: “Is the secret number on card #1? On #2? On #3? On #4? On #5?” After your friend has answered each question, you can say what the secret number is. Before you learn the “magic trick”, can you figure it out?
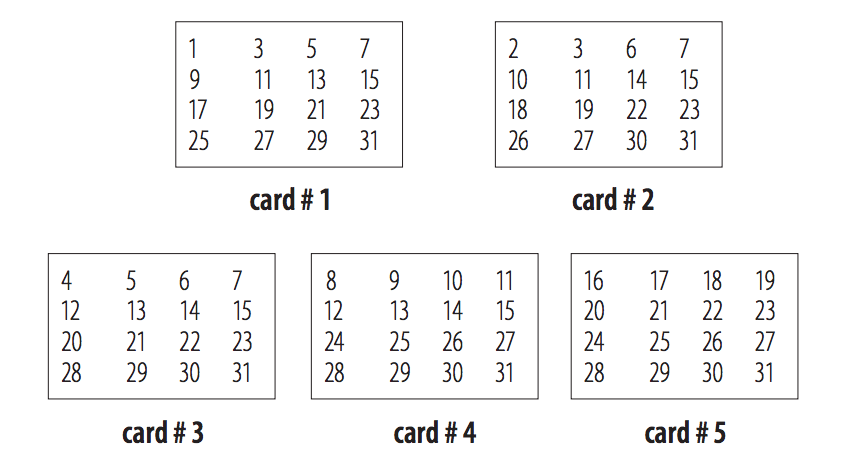
Kids love doing this activity. After they learn the “magic trick”, they become a magician themselves, who can read people’s minds. Isn’t it empowerment? Believe it or not, I tried to figure out the “magic trick” myself during a visit to the emergency department of Hospital for Sick Children with my four-year-old daughter. Blame Mr. Cornwall!
Math is a truly intriguing subject. I hope to help children develop a favourable attitude toward it and have loads of fun along the way.
Answers
Example 1: K = 7.
Example 2: P/T = R/S.
Example 3: F = 85.
Example 4: K = 11.
Example 5: There were 75 physicians at the luncheon.
Example 6:
..4369
..726
+5629
10724
..4369
..826
+5629
10824
Example 7: 9/12 + 5/34 + 7/68.
Example 8: The dimensions of the new rectangle are: 34 × 29.
Example 9: Remember the first numbers on those cards for which your friend answers ”yes” and then add them up. The sum is your friend’s secret number.
ABOUT THE AUTHOR
Gu Zhenzhen
Gu Zhenzhen, an occasional teacher, works for the Toronto District School Board.
This article is from Canadian Teacher Magazine’s Jan/Feb 2016 issue.
